As a student of Physics (believe in empiricism(~60%) and in rationalism(~40%)) I truly love Mathematical and Theoretical Biology, Systems Biology, Statistical Mechanics of Biological Systems (equilibrium and non-equilibrium) and Physics of Complex Systems: The Organizing Principles of Nature. I want to understand the philosophical connections among Mathematical and Theoretical Biology (Mathematical framework of Biological Designs, working principles as a system point of view and Dynamics), Statistical Mechanics( "The fundamental theory of a large number of agents"), & Non-Linear Dynamics. Biology is quite different than the Physical world. Studying Biology is like a thriller for Physics and Mathematics students. Though all active things obey the laws of Physics. But we can't understand their proper behaviour. Now, the question is can we propose some assumptions with respect to rigorous biological experimental data analysis (with a little bit of intuition)and computational, mathematical or quantitative models to understand this Complex World? Applied Mathematicians and Physicists didn't understand Biology properly(It's really complex!!), otherwise, they grabbed it. There is an enormous number of open questions in Biology.

 

It's really a challenging task.

 

Small overview of Complex Systems:-

 

"The elementary entities of science X obey the laws of science Y.But this hierarchy does not imply that science X is “just applied Y.” At each stage entirely new laws, concepts, and generalizations are necessary, requiring inspiration and creativity to just as great a degree as the previous one. Like

 

                              X                                           Y

         Many-Body Physics              Elementary Particle Physics

         Chemistry                              Many-Body Physics                

         Molecular Biology                Chemistry 

         Cell Biology                            Molecular Biology

                 .                                             .

                 .                                             .                 

                 .                                             .

          Psychology                            Physiology

 

Psychology is not applied biology, nor is biology applied chemistry.

But the problem is the components of complex systems are much more complicated than the simple particles of conventional statistical mechanics."

                                                                       - P.W.Anderson(Science, New Series, Vol. 177, No. 4047. (Aug. 4, 1972), pp. 393-396.)

 

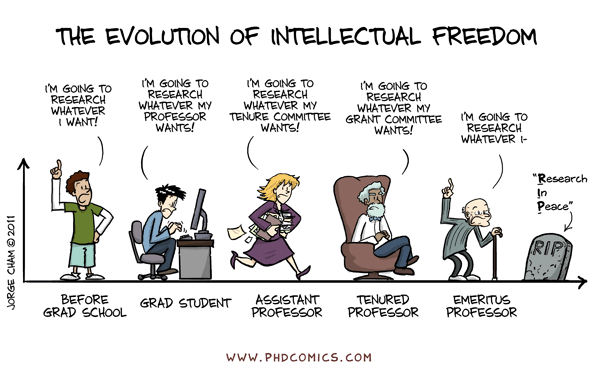
One of my favourite cartoons ------------------------------------

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

     

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Interdisciplinary Connections:-

 

     Stat. Mech. & App. Math------> In Silico------------->  

              |                                                                                  |

              |                                                                                  |

              |                                                                                  |

               <--------------- Biological Data<---------------

 

                         if   "Predictions ~ Experimental Data" 

                         then do "Speculation & make the theory"

                         else if "change assumptions and go to the loop"

                         end if

​

​

Funny and amazing Quotes:-

 

1) “With thermodynamics, one can calculate almost everything crudely; with kinetic theory, one can calculate fewer things, but more accurately; and with statistical mechanics, one can calculate almost nothing exactly.”

— Eugene Wigner (date) [27]

 

2) “A theory is the more impressive the greater the simplicity of its premises, the more different kinds of things it relates, and the more extended its area of applicability. Therefore the deep impression that classical thermodynamics made upon me. It is the only physical theory of universal content which I am convinced will never be overthrown, within the framework of applicability of its basic concepts.”

— Albert Einstein, Autobiographical Notes (c. the 1940s) 

 

3) “Thermodynamics is a funny subject. The first time you go through it, you don't understand it at all. The second time you go through it, you think you understand it, except for one or two small points. The third time you go through it, you know you don't understand it, but by that time you are so used to it, it doesn't bother you anymore.”

 

4)"The beautiful thing about the research and about the science is that there are always more questions to ask, the job is never done, it's quite depressing anyway but it's fantastic as well" by Derek Moulton (Associate Professor in Mathematical Biology in the Mathematical Institute at the University of Oxford )

 

My quotes and my thinking:-

 

1) "If there is COMPLEXITY, there is beauty(except HUMAN BEHAVIOR).We don't understand it properly, hope we can understand it in future. That's NATURE."

​

2)"Questions are questions.There are no bad, fool or good questions at all.The questions are moreover in the world are like 'How','Why','What', but 'Why' questions (means the causal effect) are rather difficult to answer in the aspect of Science or Philosophy".

​

3)"I hate the 'Theory of Deductions' to understand human nature."

​

4)"Sometimes we find our life is completely Deterministic, but rather the life where everything is Stochastic and memoryless, it will be better."

​

5)"In my early days I thought that asking questions was everything in Science, but now I understand that finding solutions to that questions are really harder than asking questions".

 

PHYSICISTS' ARROGANCE about other subjects (which is not good):-

"One conclusion... is that physics research student is apt to overestimate his understanding of things in general.Possibly we have been quietly brainwashing him unconsciously of course, into the belief that, because physics is the fundamental science, therefore with his understanding of physics he is able to cope with other sciences without further education.This unconscious arrogance is not confined to  research students. Some years ago, while taking part in a BBC panel discussion of scientific questions asked by listeners, I saw fit to contribute my pennyworth of knowledge on some biological topic.A Biologist present remarked ' We used to have a phrase about "Old wives' tales"; nowadays we just refer to "What every Physicist knows"."

---It was written by "A. B. Pippard. Reconciling physics with reality (Inaugural lecture). Cambridge: Cambridge University Press, 1971(page 18).

 

My favourite inspirational blog for playing, learning and understanding mathematics is this ( whenever I need motivation or feeling depressed, I always read this)

 

Does one have to be a genius to do maths?

by Terrence Tao

 

Better beware of notions like genius and inspiration; they are a sort of magic wand and should be used sparingly by anybody who wants to see things clearly. (José Ortega y Gasset, “Notes on the novel”) . Does one have to be a genius to do mathematics? The answer is an emphatic NO. In order to make good and useful contributions to mathematics, one does need to work hard, learn one’s field well, learn other fields and tools, ask questions, talk to other mathematicians, and think about the “big picture”. And yes, a reasonable amount of intelligence, patience, and maturity is also required. But one does not need some sort of magic “genius gene” that spontaneously generates ex nihilo deep insights, unexpected solutions to problems, or other supernatural abilities. The popular image of the lone (and possibly slightly mad) genius – who ignores the literature and other conventional wisdom and manages by some inexplicable inspiration (enhanced, perhaps, with a liberal dash of suffering) to come up with a breathtakingly original solution to a problem that confounded all the experts – is a charming and romantic image, but also a wildly inaccurate one, at least in the world of modern mathematics. We do have spectacular, deep and remarkable results and insights in this subject, of course, but they are the hard-won and cumulative achievement of years, decades, or even centuries of steady work and progress of many good and great mathematicians; the advance from one stage of understanding to the next can be highly non-trivial, and sometimes rather unexpected, but still builds upon the foundation of earlier work rather than starting totally anew. (This is, for instance, the case with Wiles‘ work on Fermat’s last theorem, or Perelman‘s work on the Poincaré conjecture.) Actually, I find the reality of mathematical research today – in which progress is obtained naturally and cumulatively as a consequence of hard work, directed by intuition, literature, and a bit of luck – to be far more satisfying than the romantic image that I had as a student of mathematics being advanced primarily by the mystic inspirations of some rare breed of “geniuses”. This “cult of genius” in fact causes a number of problems, since nobody is able to produce these (very rare) inspirations on anything approaching a regular basis, and with reliably consistent correctness. (If someone affects to do so, I advise you to be very sceptical of their claims.) The pressure to try to behave in this impossible manner can cause some to become overly obsessed with “big problems” or “big theories”, others to lose any healthy scepticism in their own work or in their tools, and yet others still to become too discouraged to continue working in mathematics. Also, attributing success to innate talent (which is beyond one’s control) rather than effort, planning, and education (which are within one’s control) can lead to some other problems as well. Of course, even if one dismisses the notion of genius, it is still the case that at any given point in time, some mathematicians are faster, more experienced, more knowledgeable, more efficient, more careful, or more creative than others. This does not imply, though, that only the “best” mathematicians should do mathematics; this is the common error of mistaking absolute advantage for comparative advantage. The number of interesting mathematical research areas and problems to work on is vast – far more than can be covered in detail just by the “best” mathematicians, and sometimes the set of tools or ideas that you have will find something that other good mathematicians have overlooked, especially given that even the greatest mathematicians still have weaknesses in some aspects of mathematical research. As long as you have education, interest, and a reasonable amount of talent, there will be some part of mathematics where you can make a solid and useful contribution. It might not be the most glamorous part of mathematics, but actually, this tends to be a healthy thing; in many cases, the mundane nuts-and-bolts of a subject turn out to actually be more important than any fancy applications. Also, it is necessary to “cut one’s teeth” on the non-glamorous parts of a field before one really has any chance at all to tackle the famous problems in the area; take a look at the early publications of any of today’s great mathematicians to see what I mean by this. In some cases, an abundance of raw talent may end up (somewhat perversely) to actually be harmful to one’s long-term mathematical development; if solutions to problems come too easily, for instance, one may not put as much energy into working hard, asking dumb questions, or increasing one’s range, and thus may eventually cause one’s skills to stagnate. Also, if one is accustomed to easy success, one may not develop the patience necessary to deal with truly difficult problems. Talent is important, of course; but how one develops and nurtures it is even more so. It’s also good to remember that professional mathematics is not a sport (in sharp contrast to mathematics competitions). The objective in mathematics is not to obtain the highest ranking, the highest “score”, or the highest number of prizes and awards; instead, it is to increase understanding of mathematics (both for yourself, and for your colleagues and students), and to contribute to its development and applications. For these tasks, mathematics needs all the good people it can get.

Further reading:

• “How to be a genius“, David Dobbs, New Scientist, 15 September 2006. [Thanks to Samir Chomsky for this link.]

• “The mundanity of excellence“, Daniel Chambliss, Sociological Theory, Vol. 7, No. 1, (Spring, 1989), 70-86. [Thanks to John Baez for this link.]